Difference between revisions of "Folklore"
m (→Various) |
(→Pascal's Triangle) |
||
| Line 77: | Line 77: | ||
== Numers & Patterns == | == Numers & Patterns == | ||
=== Pascal's Triangle === | === Pascal's Triangle === | ||
| + | An older version of the BoC website briefly had some black-and-white drawings on a page, which contained Pascal's Triangle. Alas, the page at [http://web.archive.org/web/20010606214250/http://www.boardsofcanada.com/misc.phpl web.archive.org] is dead. | ||
| + | |||
| + | Some of the pictures that were accessible on that page (and reproduced below) have interesting references that might not be apparent at first glance. Putting his interest in mathematics to use, [DC] submitted the following section about the images; he adds that, while he is interested in maths, and the mathematical patterns to be found in nature, he does not personally believe in the merits of numerology. As former maintainer of this site, he requests that that section, below, be left just as it is as his own lasting personal contribution to the site. | ||
| + | |||
| + | Firstly, there's [http://mathworld.wolfram.com/PascalsTriangle.phpl Pascal's Triangle] itself. Each number is the sum of the two diagonally above it. | ||
| + | If you start at the top and follow it down diagonally: | ||
| + | * the first column consists only of 1's (a single zero-dimensional point), | ||
| + | * the next has the positive integers 1,2,3,.... (dots arranged in a 1D line), | ||
| + | * then triangular numbers: 1,3,6,10,15,21,... (dots arranged in 2D triangles), | ||
| + | * then the tetrahedral/pyramidal numbers : 1,4,10,20,35,56... (dots arranged in 3D tetrahedrons); | ||
| + | * then: 1,5,15,35,70,126... (dots arranged in 4D '4-simplex' arrangements); | ||
| + | and so in, into ever higher-dimensional analogues of the triangle. | ||
| + | |||
| + | Another example - tossing coins: take the row "1,4,6,4,1". If you toss a coin 4 times, the chances of getting 0,1,2,3, and 4 heads (or tails) are, respectively: 1/16, 4/16, 6/16, 4/16, 1/16. If you toss it 5 times, the chances of 0,1,2,3,4,5 heads (or tails) are: 1/32, 5/32, 10/32, 10/32, 5/32, 1/32 (from the next row). And so on. | ||
| + | |||
| + | The total of the numbers in each row is a power of two. There are many more relations - a whole page could be devoted to Pascal's Triangle alone - but I'll let readers pursue this themselves. There's a Dr Math article on it, and another [http://ptri1.tripod.com/ site devoted to Pascal's Triangle] even explains how you can get the Fibonacci numbers from it, among other things. | ||
| + | |||
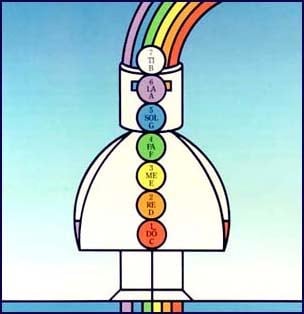
=== Various Math Related Pictures === | === Various Math Related Pictures === | ||
Revision as of 18:56, 23 August 2008
Contents
Artwork Allusions
Icredible String Band
Allusions in the artwork. Particularly referring to the Incredible String Band, for whom BoC have a great deal of respect, and whom they apparently see from time to time. The lyrics section reveals that they have sampled the ISB occasionally; there's the old track "ithcus sound", a possible reference to the ISB track "ithkos". There's a general similarity in mood between Geogaddi and such ISB songs as "Waltz of the new moon" (HBD). It has also been observed that the title "Geogaddi" might in part refer to the ISB song "koeeaddi there" (HBD again).
One interviewer comments that the front covers of the ISB's "The Hangman's Beautiful Daughter" and BoC's "Music Has The Right To Children" would make "a nice pair":
However, but there is a far more striking similarity between the back cover of the ISB album, and some of the pictures of BoC available elsewhere; if this is any more than coincidence, and it may be no more than that, it makes a nice tribute to the ISB:
The next pair aren't all that similar, except in their mood, but here they are anyway:
Geogaddi Cover
[Roger B] has pointed out something about the cover art of the Geogaddi album: In the scene in the film "The Wicker Man" that features dancing around a maypole, a figure is seen in silhouette (by his shadow on the grass). The pose is very similar to that in the picture used to make the album cover. (Thanks, Roger). Below, is a frame from that film. To the right, the photo on which the Geogaddi cover is based (the picture was taken by Peter Iain Campbell, as is noted on the back of the album).
However, [Tonamel Rhysthal] has another suggestion, that the pose mirrors that of Leonardo da Vinci's famous "Vitruvian Man". Wikipedia, always a good resource, has an article on Vitruvian Man. The link between this figure and the relation between art and mathematics fits in very well with some of the other images BoC had on their site (see below), and also with one of the themes of Geogaddi (religious iconography and geometry).
My Bloody Valentine
The following information has been given by [7seals]. The front covers of both albums. Note the very similar font used (Bauhaus). Faces obscured. (in Music Has the Right to Children, it seems artificial whereas in isn't anything it seems to be intentionally caused by overexposure).
The inside front covers.
The inserts. This one on the left is surely a representation of Michael Sandison and Marcus Eoin of Boards of Canada. On the right is a band photograph of My Bloody Valentine.
The back covers of the albums.
[Khov] made a post on the watmm forum about one of the small artwork pictures on the campfire headphase cover which obviously has been taken from this 1979 yearbook picture.
The Campfire Headphase
[Khov] made a post on the watmm forum about one of the small artwork pictures on The Campfire Headphase cover which obviously has been taken from [this 1979 yearbook picture].
Trans Canada Highway Cover
[Damien] made a post on the old guestbook about the striking resemblance between the Trans Canada Highway cover and a 1977 Dodge operation manual. No coincidence.
Various
[DC] stumbled across this purely by accident: one of the little images in the artwork of the In a Beautiful Place out in the Country single comes from here (where the image is explained).
Numers & Patterns
Pascal's Triangle
An older version of the BoC website briefly had some black-and-white drawings on a page, which contained Pascal's Triangle. Alas, the page at web.archive.org is dead.
Some of the pictures that were accessible on that page (and reproduced below) have interesting references that might not be apparent at first glance. Putting his interest in mathematics to use, [DC] submitted the following section about the images; he adds that, while he is interested in maths, and the mathematical patterns to be found in nature, he does not personally believe in the merits of numerology. As former maintainer of this site, he requests that that section, below, be left just as it is as his own lasting personal contribution to the site.
Firstly, there's Pascal's Triangle itself. Each number is the sum of the two diagonally above it. If you start at the top and follow it down diagonally:
- the first column consists only of 1's (a single zero-dimensional point),
- the next has the positive integers 1,2,3,.... (dots arranged in a 1D line),
- then triangular numbers: 1,3,6,10,15,21,... (dots arranged in 2D triangles),
- then the tetrahedral/pyramidal numbers : 1,4,10,20,35,56... (dots arranged in 3D tetrahedrons);
- then: 1,5,15,35,70,126... (dots arranged in 4D '4-simplex' arrangements);
and so in, into ever higher-dimensional analogues of the triangle.
Another example - tossing coins: take the row "1,4,6,4,1". If you toss a coin 4 times, the chances of getting 0,1,2,3, and 4 heads (or tails) are, respectively: 1/16, 4/16, 6/16, 4/16, 1/16. If you toss it 5 times, the chances of 0,1,2,3,4,5 heads (or tails) are: 1/32, 5/32, 10/32, 10/32, 5/32, 1/32 (from the next row). And so on.
The total of the numbers in each row is a power of two. There are many more relations - a whole page could be devoted to Pascal's Triangle alone - but I'll let readers pursue this themselves. There's a Dr Math article on it, and another site devoted to Pascal's Triangle even explains how you can get the Fibonacci numbers from it, among other things.